wishIwasSalludon
leave this place behind
- Joined
- Nov 9, 2023
- Posts
- 17,807
- Reputation
- 24,012
In continuum mechanics, the Cauchy stress tensor(symbol
, named after Augustin-Louis Cauchy), also called true stress tensor[1] or simply stress tensor, completely defines the state of stress at a point inside a material in the deformed state, placement, or configuration. The second order tensor consists of nine components
and relates a unit-length direction vector e to the traction vector T(e) across an imaginary surface perpendicular to e:
[a]
The SI base units of both stress tensor and traction vector are newton per square metre (N/m2) or pascal (Pa), corresponding to the stress scalar. The unit vector is dimensionless.
The Cauchy stress tensor obeys the tensor transformation law under a change in the system of coordinates. A graphical representation of this transformation law is the Mohr's circle for stress.
The Cauchy stress tensor is used for stress analysisof material bodies experiencing small deformations: it is a central concept in the linear theory of elasticity. For large deformations, also called finite deformations, other measures of stress are required, such as the Piola–Kirchhoff stress tensor, the Biot stress tensor, and the Kirchhoff stress tensor.
According to the principle of conservation of linear momentum, if the continuum body is in static equilibrium it can be demonstrated that the components of the Cauchy stress tensor in every material point in the body satisfy the equilibrium equations (Cauchy's equations of motion for zero acceleration). At the same time, according to the principle of conservation of angular momentum, equilibrium requires that the summation of momentswith respect to an arbitrary point is zero, which leads to the conclusion that the stress tensor is symmetric, thus having only six independent stress components, instead of the original nine. However, in the presence of couple-stresses, i.e. moments per unit volume, the stress tensor is non-symmetric. This also is the case when the Knudsen number is close to one,
, or the continuum is a non-Newtonian fluid, which can lead to rotationally non-invariant fluids, such as polymers.
There are certain invariants associated with the stress tensor, whose values do not depend upon the coordinate system chosen, or the area element upon which the stress tensor operates. These are the three eigenvalues of the stress tensor, which are called the principal stresses.
@cromagnon @ey88 @moredatesmorerapes
| Cauchy stress tensor | |
|---|---|
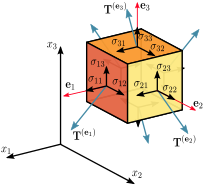 Components of stress in three dimensions | |
| Common symbols | σ |
| SI unit | pascal (Pa) |
| Other units | Pound per square inch(psi), bar |
| In SI base units | Pa = kg⋅m−1⋅s−2 |
| Behaviour under coord transformation | tensor |
| Dimension |
The SI base units of both stress tensor and traction vector are newton per square metre (N/m2) or pascal (Pa), corresponding to the stress scalar. The unit vector is dimensionless.
The Cauchy stress tensor obeys the tensor transformation law under a change in the system of coordinates. A graphical representation of this transformation law is the Mohr's circle for stress.
The Cauchy stress tensor is used for stress analysisof material bodies experiencing small deformations: it is a central concept in the linear theory of elasticity. For large deformations, also called finite deformations, other measures of stress are required, such as the Piola–Kirchhoff stress tensor, the Biot stress tensor, and the Kirchhoff stress tensor.
According to the principle of conservation of linear momentum, if the continuum body is in static equilibrium it can be demonstrated that the components of the Cauchy stress tensor in every material point in the body satisfy the equilibrium equations (Cauchy's equations of motion for zero acceleration). At the same time, according to the principle of conservation of angular momentum, equilibrium requires that the summation of momentswith respect to an arbitrary point is zero, which leads to the conclusion that the stress tensor is symmetric, thus having only six independent stress components, instead of the original nine. However, in the presence of couple-stresses, i.e. moments per unit volume, the stress tensor is non-symmetric. This also is the case when the Knudsen number is close to one,
There are certain invariants associated with the stress tensor, whose values do not depend upon the coordinate system chosen, or the area element upon which the stress tensor operates. These are the three eigenvalues of the stress tensor, which are called the principal stresses.
@cromagnon @ey88 @moredatesmorerapes
